THE LAW OF ONE PRICE, PURCHASING POWER PARITY AND EXCHANGE
RATES

 

Conversion standard financial matters is loaded up with puzzles. The resource approach has
fizzled and without it most open-economy models are based on sand.
The tried and true way of thinking rejects the Law of One Cost and perspectives Buying
Power Equality as helpful, best case scenario, over the long haul. We show interestingly how
perceiving contrasts between retail, discount and sale markets, and
perceiving that exchange includes time on the way, settles the riddles and
gives a hypothesis of trade rates utilizing closeout markets for resources and
products. We additionally reestablish the Law of One Cost and Buying Power
Equality to the situation with “not dismissed”. THE LAW OF ONE PRICE and PPP.

1. Introduction for THE LAW OF ONE PRICE and PPP

With the caveat that it might be useful in the long run, conventional
exchange-rate economics rejects Purchasing Power Parity. When applied to
commodity markets, it also rejects the Law of One Price. We argue that
those rejections are unwarranted because they use seriously flawed
“semantic rules”. After describing the flaws with those tests, we suggest
appropriate tests and propose a theory of exchange rates using auction
prices that combines an appropriate version of Purchasing Power Parity with
Covered Interest Parity.
To clarify the discussion, we use the acronyms LOP and PPP to refer to
the theories or ideas behind the Law of One Price and Purchasing Power
Parity. CLOP and CPPP include the “semantic rules” that conventional tests
use to make those theories operational. ALOP and APPP use the more
appropriate semantic rules suggested here.

The paper is organized as follows: Section 2 discusses the role of
semantic rules in testing theories including the LOP and PPP. Section 3
defines what we mean by the LOP and PPP. Section 4 critically reviews the
conventional tests of the LOP and PPP and suggests more appropriate
semantic rules. Combining CIP with APPP, Section 5 develops a new
approach to the determination of spot exchange rates based on effective
arbitrage in auction markets for assets and commodities. THE LAW OF ONE PRICE and PPP.

2. Semantic Rules for THE LAW OF ONE PRICE and PPP

We can lack an abundance of trust in dismissing the Trim, PPP or some other
hypothesis than we have in the semantic principles used to test them.1
Assuming we acknowledge
the thoughts behind the ALOP and APPP, then, at that point, we ought to lack trust in
the semantic guidelines used to date to test the Trim and PPP. All things considered, those
tests are uninformative and the Hack and PPP ought to be reestablished to “not
dismissed”.
The accompanying outlines the job of semantic principles in testing theories.2

Let a→b mean “on the off chance that a, b”. a→b rejects that an is “valid” and that b isn’t
valid, i.e., n(a˄nb), which thusly suggests that either an isn’t correct or b is valid,
for example na˅b. The important point is that a→b is “valid” when an is “bogus”
whether or not b is “valid” or “false”.
Allow T to address the Cut, PPP or any hypothesis and S the relating
semantic principles. To be experimentally significant, a portion of the terms in T must
be connected to things we can gauge. THE LAW OF ONE PRICE and PPP.

An Educational explanation for THE LAW OF ONE PRICE and PPP

An Educational explanation that 100 heavenly messengers can fit on the top of a pin is
an illustration of an explanation that isn’t experimentally significant on the grounds that there
is no chance, even in head, to quantify the quantity of heavenly messengers.
One method for communicating the sensible design engaged with testing a hypothesis is
as follows: T→ S→p→q where p→q addresses some testable
ramifications of joining T and S. Note that dismissing the testable
suggestions doesn’t, without help from anyone else, reject T. On the off chance that S is “bogus”, S→(p→q) is
“valid” in any event, when the proof oddballs (p→q). Subsequently, T→ (S→p→q) is
“valid” and the proof doesn’t dismiss T.
Hypotheses compel semantic principles. Take the law of gravity. Dropping a
feather and an iron ball from the inclining pinnacle of Pisa doesn’t dismiss the
regulation since it requires a vacuum. Dropping an iron ball on the moon where
it doesn’t advance rapidly at 32 feet each second doesn’t dismiss the law of gravity
since it relies upon mass.

This paper says that we ought to lack trust in the semantic
rules utilized by the CLOP to date to dismiss the Cut, and the CPPP to dismiss PPP
since they are conflicting with the speculations. Thus, the Hack and PPP
ought to be reestablished to “not dismissed”. THE LAW OF ONE PRICE and PPP.

3. Definitions for THE LAW OF ONE PRICE and PPP

Definitions of the LOP and PPP in dictionaries, encyclopedias and
Wikipedia usually include some semantic rules. The following definitions of
the LOP and PPP are based on those definitions, but without any semantic
rules.

1. LOP for THE LAW OF ONE PRICE and PPP

The following is our definition for the LOP: “Arbitrage works to equate
prices for the same good in different locations.” For examples of definitions
like this one, see Sarno and Taylor (2002a, 52) and Black, Hashimzade and
Myles (2012, 234). When we refer to the LOP we mean that theory or core
idea.

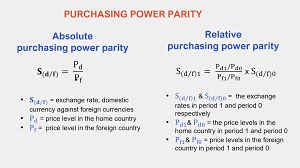
2. PPP for THE LAW OF ONE PRICE and PPP

There are a few renditions of PPP. The utility rendition for instance says
that $100 ought to purchase a similar measure of “utility” at home and abroad. In any case
the rendition in view of the Trim utilized here is by a long shot the most widely recognized. It is the
one tracked down in many course books and articles as well as in broadened conversations
of trade rates like Isard (1995) and Sarno and Taylor (2002a). In the event that the Hack
holds for each great, then the swapping scale should rise to the “homegrown cost
level” partitioned by the “unfamiliar cost level” where both “cost levels” have the
same loads. THE LAW OF ONE PRICE and PPP.
Thusly, coming up next is our meaning of PPP: “Exchange works to
compare trade rates with proportions of cost levels for the two nations where
both cost levels have similar loads.”
Neither one of the speculations is functional. The following area considers the semantic
rules used to make them functional, beginning with CLOP.

4. Testing for THE LAW OF ONE PRICE and PPP

This part fundamentally audits how ordinary swapping scale
financial aspects has utilized improper semantic guidelines to test the Cut and PPP. It
likewise proposes more fitting semantic principles. It starts with the CLOP.

1. CLOP for THE LAW OF ONE PRICE and PPP

This subsection depicts the ordinary way to deal with testing the
Trim, what we call the CLOP. Disregarding edges, Rogoff (1996, 649)
depicts an ordinary somewhat functional rendition of the Trim for
item showcases as follows: Pi = EP* where E is the homegrown cost of
unfamiliar trade while Pi and P* are costs for a similar item in two
various nations. It is obvious from the setting that E, Pi and P* are spot
costs.

As it stands, Pi = EP* is not operational. E, Pi and P* are purely
theoretical terms with no link to things we can observe. “Semantic rules”
establish those links. The relevant conventional literature like Rogoff (1996)
and the articles cited in the following paragraph link E to spot auction
markets while linking Pi and P* to spot retail markets.
The conventional view that the LOP fails rests largely on influential
articles like Engel and Rogers (1996), Asplund and Friberg (2001), and
Parsley and Wei (2001).
They all link Pi and P* to current retail prices and E
to a current auction exchange rate.
These conventional semantic rules seriously bias tests of the LOP and
also help create most of the foreign-exchange puzzles discussed in Section 6.

2. ALOP for THE LAW OF ONE PRICE and PPP

This subsection talks about the defects in the ordinary trial of the Cut
what’s more, gives a superior method for testing the hypothesis. We call it the closeout and
exchange form of the Hack, or ALOP.
The first serious defect in quite a while of the Hack like those by
Engel and Rogers (1996), Asplund and Friberg (2001), and Parsley and Wei
(2001) is that they disregard the distinction among retail and closeout markets.4
Whether monetary or product, there are three significant sorts of business sectors:
retail, discount and sale.
In retail product markets, bread is exchanged by the portion, in discount
markets by the truck load. In sell off business sectors wheat is exchanged by the boat
load. Whether monetary or item, exchange and data costs per
dollar exchanged are most noteworthy in retail where the amount exchanged is least and
least in sell off business sectors where the amount exchanged is most noteworthy. The
generally significant expenses in retail showcases assist with making sense of the shortfall of exchange and
exchange in global retail showcases delineated.

Customary trial of the Cut use ware costs from retail for THE LAW OF ONE PRICE and PPP

Customary trial of the Cut use ware costs from retail markets, however there is no exchange or even exchange between retail markets.
one purchases shoes from Macy’s in New York and afterward offers them to Marshall
Fields in Chicago. Somebody from Chicago could purchase shoes in London, Paris
or on the other hand New York and bring them back home, however that is not really “exchange”.
The shortfall of exchange between retail showcases doesn’t imply that they
are not connected. A firm creating shoes in Milan offers those shoes to retailers
in Chicago, London, Paris and New York. Kansas ranches produce wheat that is
exchanged sell off business sectors and that is processed into the flour that pastry kitchens in
Chicago, London, Paris and New York use to prepare bread. Retail advertises are
connected, yet the connections are frail, backhanded and work gradually.

The second serious defect is an immediate consequence for THE LAW OF ONE PRICE and PPP

The second serious defect is an immediate consequence of the first. Ordinary
trial of the Cut blend retail ware costs in with closeout trade rates.

This blend adds to a few of the riddles in open-economy
macroeconomics talked about in Area 6 since it influences customary tests
of PPP.
The third serious defect in traditional trial of the Hack like those by
Engel and Rogers (1996), Asplund and Friberg (2001), and Parsley and Wei
(2001) is that they utilize current costs and trade rates when ware
exchange includes time on the way. Time on the way infers that one can’t buya ware in one area and all the while sell it in another area
risk free as expected by exchange.
As far as we could possibly know, Benninga and Protopapadakis (1988)
were quick to bring up the significance of time on the way for the Trim, yet
they focus on what it means for spot cost differentials.7
This paper contends
that time on the way changes how we ought to ponder the actual Hack. In
item advertises, the Hack applies to advance costs and trade rates,
not spot costs and trade rates.

Disregarding limits and loan for THE LAW OF ONE PRICE and PPP

The rationale behind the Trim applying to advance costs and trade
rates as opposed to detect is as per the following: Disregarding limits and loan costs for
effortlessness, compelling homegrown exchange compares the spot and forward costs
of W in Bay Ports while powerful global exchange likens the forward
cost in Rotterdam times the forward dollar cost of the euro with the spot
cost in Bay ports. With time on the way, successful exchange and the Trim
consequently suggests that ($/€)90(€/W)90 = ($/W)90 in light of the fact that it suggests that ($/€)90(€/W)90
what’s more ($/W)90 both equivalent ($/W)0.

Comparative contentions don’t have any significant bearing to detect rates in light of the fact that the element of
one spot cost generally contrasts from the element of the forward cost suggested
by global exchange. For instance, actually overlooking limits and
loan costs for straightforwardness, that’s what compelling global exchange infers
($/€)90(€/W)90 = ($/W)0 where both are in dollars, while compelling homegrown
exchange in Rotterdam suggests that (€/W)90 = (€/W)0 where both are in euros.

($/W)0 doesn’t rise to (€/W)0 on the grounds that ($/€)90(€/W)90 doesn’t approach (€/W)90.
Adding loan costs and limits doesn’t change the way that ($/€)90(€/
W)90 is in dollars while (€/W)90 is in euros.
Subsections 4.2.1 to 4.2.3 give an illustration of how this functions with
financing costs and edges. W is a specific assortment of wheat with explicit
protein content and indicated values for the wide range of various qualities typically
remembered for agreements to trade W in a sale market. For straightforwardness,
there is no verifiable re-visitation of holding W. Firms like Bunge Ltd. furthermore, Cargill Inc.
are as ready to hold a lot of W spot concerning own a case on that wheat in 90
days. Firms are likewise as ready to hold a case on a lot of wheat in a Bay
port as in Rotterdam. Counting such expenses or returns would just confuse
the limits talked about in Subsection 4.2.3.

Neighborhood intertemporal harmony for THE LAW OF ONE PRICE and PPP

4.2.1. Neighborhood intertemporal harmony. There is neighborhood intertemporal
harmony when it is difficult to create sans risk gains by purchasing spot and
selling forward or the inverse. Harmony likewise avoids misfortunes. When
joined with global intertemporal harmony, this neighborhood balance
produces trial of the Trim utilizing forward costs and forward trade rates
from sell off business sectors.

The US is the nation of origin for THE LAW OF ONE PRICE and PPP

The US is the nation of origin. ($/W)0 is the spot cost of W in
U.S. Bay ports and ($/W)90 is the 90-day forward cost. CC$90($/W)90 is the
cost in later dollars of conveying W forward 90 days in Bay ports. It is
exogenous in light of the fact that W is only one of a wide assortment of grains conveyed forward.

I is the 90-day financing cost in the U.S. It is exogenous in light of the fact that the
getting and loaning related with exchange W is a miniscule piece of the
significant capital market. For effortlessness, the conversation disregards the distinction
among bid and ask costs, and acquiring and loaning rates. They would
simply muddle the limits examined underneath.
Eq. (1) is one method for composing neighborhood balance.
[($/W)90 – CC$90($/W)90]/(1+ I) = ($/W)0
(1)
Subsequent to representing conveying costs, the current worth of W conveyed forward
approaches the spot worth of W.

In the case of, beginning in harmony, ($/W)0 falls, ($/W)90 rises, conveying costs fall
or on the other hand financing costs fall, there are “without risk” profits.8
[($/W)90 –
CC$90($/W)90]/(1+i) is more prominent than ($/W)0. Arbitragers purchase low and sell high.

They acquire W($/W)0 spot dollars

They acquire W($/W)0 spot dollars, which they reimburse with W($/W)0(1+i) future
dollars, and purchase W spot. They sell W forward and convey it forward to meet
their future responsibility. [($/W)90 – 0CC$90($/W)90]/(1+ I) – ($/W)0 is the riskfree benefit. Spot buys raise ($/W)0 and forward deals lower ($/W)90 until
exchange reestablishes harmony.
In the case of, beginning in harmony, ($/W)0 rises, ($/W)90 falls, conveying costs rise or
financing costs rise, conveying W forward produces misfortunes. [($/W)90 –
CC$90($/W)90]/(1+ I) is not exactly ($/W)0. Arbitragers answer by selling high
what’s more, purchasing low. They “get” spot W and undercut it, contribute the returnsand buy forward.9
Selling spot lowers ($/W)0 and buying forward raises
($/W)90, but it does not fully restore eq. (1) unless the cost of selling short is
zero. Let εW represent the cost of borrowing W for 90 days over and above
the interest rate. If ε is zero, as long as [($/W)90 – 0CC$90($/W)90] < ($/W)

Free profit by selling spot and buying forward

arbitragers make a risk-free profit by selling spot and buying forward. If ε
is positive, selling short produces a risk-free profit only as long as [($/W)90 –
0CC$90($/W)90] < ($/W)0(1+ i + ε).
For simplicity, the discussion beyond this point ignores ε because ε just
complicates the thresholds discussed below. How well auction markets
respond to such shocks and restore equilibrium is an empirical issue that
needs to be addressed more fully. What follows assumes that eq. (1) holds.
Eq. (1) can be written as follows:

($/W)90[1 – 0CC$90] = ($/W)0(1+ I)
(1′) Subsequent to representing the conveying costs, the future worth of
present wheat rises to the future worth of future wheat.
Comparative exchanges produce comparable equilibria in Rotterdam. The
documentation for Rotterdam is as per the following: (€/W)0 is the spot euro cost of W in
Rotterdam and (€/W)90 is the forward euro cost of W in Rotterdam in 90
days. CC€90(€/W)90 is the expense in later euros of conveying W forward by 90
days in Rotterdam. It is exogenous for a similar explanation the conveying cost in
Bay ports is exogenous. i* is the 90-day euro financing cost. It is exogenous
for a similar explanation I is exogenous. Eq. (2) portrays the pertinent neighborhood
harmony in Rotterdam.

(€/W)90[1 – CC€90] = (€/W)0(1+ i*)

Full international equilibrium assumes local equilibrium.

2. International equilibrium.

Near advantage drives exchange.
See Wikipedia for a conversation of near advantage. With trade
rates exogenous, the heading of exchange for W relies upon where, in the
nonattendance of exchange, W is least expensive in a typical money.
Because of time on the way, any place W is least expensive without even a trace of exchange,
direct exchange between spot ware markets in various areas is
unthinkable, as is immediate exchange between forward business sectors of the equivalent
development in various areas. However, exchange is conceivable between t = x and t
= y, for however long y is adequately more noteworthy than x to consider time in transit.10 In
this model, x is zero and y is 90 days.

($/€)0 is the spot dollar cost of the euro and ($/€)90 is the 90-day
forward cost of the euro. (€/$)0 is the spot euro cost of the dollar and
(€/$)90 is the 90-day forward cost of the dollar. For effortlessness, the conversation
overlooks bid-ask spreads, ($/€)0 = 1/(€/$)0 and ($/€)90 = 1/(€/$)90. Trade
rates are exogenous in light of the fact that the unfamiliar trade engaged with exchanging W is
just an infinitesimal piece of the unfamiliar trade market.
TC$90(€/W)90($/€)90 is the expense in later dollars of transportation W from a Bay
port to Rotterdam while TC€90($/W)90(€/$)90 is the expense in later euros of shipping W from Rotterdam to a Gulf port.

Traded between Gulf ports and Rotterdam.

They are exogenous because W is only one of many grains traded between Gulf ports and Rotterdam. Ignoring for a moment transport costs, carrying costs and interest rates,
W flows from Gulf ports to Rotterdam when, in the absence of trade, W is
cheaper in Gulf ports, e.g., when ($/W)90 is less than ($/€)90(€/W)90.

The flows from Rotterdam to Gulf ports when (€/W)90 is less than (€/$)90($/W)90, i.e.,
when ($/W)90 is greater than ($/€)90(€/W)90. Subsection 4.2.3 discusses the
thresholds created by transport and carrying costs.
When Gulf ports have the price advantage, if W moves, it moves from
Gulf ports to Rotterdam. In that case, one way to express equilibrium is that
($/€)90(€/W)90[1 – TC$90] = ($/W)0(1+ i). The future dollar value of spot
W in a Gulf port equals the future dollar value of shipping W to Rotterdam,
selling it forward there and selling those future euros forward at ($/€)90. Note
that trade can continue from day to day in this equilibrium without any riskfree profits or avoidable losses. They become relevant when equilibria are
violated.

Exchange benefit in light

On the off chance that, beginning in balance yesterday, today ($/€)90 rises, (€/W)90 rises, ($/
W)0 falls, I falls or TC$90 falls, today there is an exchange benefit in light of the fact that
($/€)90(€/W)90[1 – 0TC$90] >($/W)0(1+i). Arbitragers acquire W($/W)0 spot
dollars which they reimburse with W($/W)0(1+i) future dollars, purchase W spot in aInlet port, transport it to Rotterdam where they sell it forward for W(€/W)90 and sell
those forward euros for forward dollars. They do this as near
at the same time as could really be expected. Buys raise ($/W)0 and deals decrease (€/W)90,
reestablishing balance.
If, beginning in balance yesterday, today ($/€)90 falls, (€/W)90 falls,
($/W)0 rises, I rises, or TC$90 rises, then ($/€)90(€/W)90[1 – TC$90] <
($/W)0(1+i). Assuming these progressions are sufficiently huge, Inlet ports might lose their
benefit and W moves from Rotterdam to Inlet ports, bringing down ($/W)0 by
bringing down ($/W)90 and raising (€/W)90.
In the event that the shock doesn’t move the benefit to Rotterdam, yet diminishes the
Bay port benefit with the goal that it no longer takes care of the net exchange costs,
exchange stops. (€/W)90 ascends as imports stop and ($/W)0 falls as commodities stop,
however, this shortfall of exchange doesn’t be guaranteed to reestablish harmony. The
conversation of limits in Subsection 4.2.3 depicts what occurs in that
case.

On the off chance that Inlet ports hold the value benefit and exchange proceeds, arbitragers
undercut spot W in Bay ports and purchase W forward in whichever forward
market is least expensive. With ε the expense of undercutting W, exchange reestablishes
harmony up to the place where ($/W)90(€/W)90[1-0TC$90] = ($/W)90[1-
0CC$90] = ($/W)0(1+i+ε). For effortlessness, the conversation underneath disregards ε,
which simply entangles the limits.

Full global balance requires

Full global balance requires nearby harmony. Utilizing the
harmony condition in Bay ports that ($/W)90[1-0CC$90] = ($/W)0(1+i),global balance with exchange from Inlet ports to Rotterdam can be
composed as follows: ($/€)90(€/W)90[1-TC$] = ($/W)90[1-CC$]. Settling that
condition for [($/W)90/(€/W)90] yields eq. (3).
[($/W)90/(€/W)90] = [($/€)90(1-TC$)]/[1-CC$]
(3) Exogenous trade rates, transport costs and conveying costs decide
relative costs in balance.
Utilizing the estimation that log(1+a) rises to a when an is little, eq. (3)
can be written in logarithmic structure as eq. (3′).
log[($/W)90/(€/W)90] = log($/€)90 – [TC$-CC$]
(3′)
Exchanges like those examined above produce equilibria for
purchasing in Rotterdam and selling in Bay ports: (€/$)90($/W)90[1-TC€] =
(€/W)0(1+i*).

Utilizing the neighborhood Rotterdam balance that (€/W)90[1-CC€] =
(€/W)0(1+i*), the global harmony that (€/$)90($/W)90[1-TC€] =
(€/W)0(1+i*) can be composed as eq. (4).
($/W)90/(€/W)90= [1-CC€]/{(€/$)90[1-TC€]} = {($/€)90[1-CC€]}/[1-TC€]
(4) In harmony, exogenous trade rates, transport costs and conveying
costs decide ($/W)90/(€/W)90.
Utilizing logarithms, eq. (4) can be composed as eq. (4′).
log[($/W)90/(€/W)90] = log($/€)90 + [TC€ – CC€]
(4′)
Eqs. (3′) and (4′) contrast by [TC$ – CC$] and [TC€ – CC€], the edges.

3. Thresholds.

To perceive how exchange costs make edges,
look at first as a world without transport costs, conveying expenses or loan fees,
in any case, with a given swapping scale. Let ($/€)90 be that rate. For ($/W)90/(€/W)90
< ($/€)90 without even a trace of exchange, Bay ports product to Rotterdam in light of the fact that in
dollars W is less expensive in Bay ports.

Trace of exchange falls

As (€/W)90 without even a trace of exchange falls or ($/€)90 without even a trace of exchange
rises, that benefit declines until it arrives where ($/W)90/(€/W)90 =
($/€)90. Exchange stops. Call that ($/W)90/(€/W)90 tipping point T.
As ($/W)90/(€/W)90 without any exchange ascends past T, the benefit
changes to Rotterdam in light of the fact that the dollar cost of W without even a trace of exchange
is currently lower in Rotterdam than in Bay ports.
Presently consider the impact of simply transport costs. For a scope of
$/€)90(€/W)90 beneath T, transport costs forestall Bay ports from sending out to
Rotterdam. Call that tipping point L where L = T(1─TC$). For
Rotterdam to commodity to Bay ports, Rotterdam’s benefit should cover its
transport costs. Call that higher tipping point U where U = T(1+TC€).

Disregarding conveying costs, U is the upper edge and L is the lower
limit. Between those limits the harmony conditions created
above don’t hold. Therefore, ($/W)90/(€/W)90 can move pretty much unreservedly
among U and L. Counting conveying costs changes U and L, yet it doesn’t
change the rationale behind limits.

This part portrays conveying and transport costs so they make
log straight limits, yet that rearrangements conceals a portion of the intricacy of
the limits. The Supplement utilizes more “practical” conveying and transport
costs.
The essential goal of Areas 4.2.1 to 4.2.3 is to clarify that,
with time on the way, the Hack holds at forward costs and trade rates, not
spot costs and trade rates.
Trade rates, loan fees and it are exogenous here to convey costs
for effortlessness. As the quantity of products exchanged increments as in PPP, they
become endogenous.

4. PPP.

Most conventional tests of Purchasing Power Parity assume, implicitly
or explicitly, that they are testing the arbitrage version adopted here that
depends on an effective Law of One Price, and, therefore, on effective
arbitrage.

CPPP

Involving the CLOP as an establishment, Rogoff (1996, 650)
portrays customary outright PPP as follows: Pi = EP*, or E = Pi/P*, where
these totals are over purchaser cost files.
The express semantic rule for
“cost” in Rogoff (1996) is that it is a purchaser, i.e., a retail, price.15 As with
the CLOP, the certain semantic decide for E is that it is a resource cost, i.e., anauction price. It is clear that these are current prices. Following
conventional views, Rogoff rejects absolute PPP in favor of the relative
version.
Like most of the literature, Rogoff skims over the changes necessary to
shift from testing the Law of One Price to testing Purchasing Power Parity.
With the Law of One Price, exchange rates and the relevant transaction costs
are often treated, implicitly or explicitly, as exogenous. With Purchasing
Power Parity, they become endogenous. The shift from exogenous to
endogenous is the same for CPPP and APPP, but it needs to be examined
more closely.

APPP

This subsection examines the defects in the customary
trial of PPP, which are equivalent to for CPPP and proposes a superior approach to
test Buying Power Equality. We call that better way the closeout and
exchange variant of PPP, or APPP.
The principal imperfection in CPPP trial of the PPP is that they use retail item
costs where there is no exchange and exchange is unimaginable. Whatever CPPP
tests test, it isn’t the PPP in view of the Hack on the grounds that the Trim depends on
endlessly exchange is unthinkable between retail advertises.
The subsequent imperfection is that CPPP tests blend retail ware costs in with
sell off trade rates. This combination causes the majority of the open-economy
puzzles talked about in Area 6.

The third defect is that CPPP

The third defect is that CPPP use spot costs to test PPP when time in
travel infers that the Cut, which is the reason for PPP here, doesn’t make a differenceto detect costs. Anything that CPPP tests test, it isn’t the PPP in view of the Hack
since the Trim depends on endlessly exchange, which requires
“synchronous” buys and deals, is beyond the realm of possibilities between spot
ware markets.
APPP suggests an alternate approach to testing PPP. Disregarding edges, let
Π(t+y) signify a homegrown container of forward sell off costs at t for t+y where
the span among t and y is sufficiently enormous to cover time on the way. In
Segment 4.2.2, ($/W)90 is such a cost. Let Π*(t+y) signify an unfamiliar container of
forward sell off costs at t for t+y with similar loads as Π(t+y). In
Segment 4.2.2, (€/W)90 is such a cost. Let F(t+y) signify the forward
swapping scale at t for t+y. In Segment 4.2.2, ($/€)90 is such a swapping scale.
Eq. (5) depicts APPP:
F(t+y) = Π(t+y)/Π*(t+y)

where the exchange rate and commodity prices are all auction prices. Unlike
CPPP, there is no reason to dismiss even short-run absolute APPP out of
hand.
There are far fewer auction commodity prices than retail commodity
prices, but there are probably more auction commodity prices than most
economists realize. In addition, unlike retail prices that are “sticky”, auction
commodity prices like auction exchange rates are, to a reasonable first
approximation, martingales. Table 1 provides a sample of such prices and a
simple test for white noise for first differences in logs.

5. ACTFX.

This section develops a theory of exchange rate determination based
on arbitrage in auction markets for both assets and commodities. It begins
with Covered Interest Parity where i(t+y) is the domestic interest rate at t
with maturity y and i*(t+y) is the foreign interest rate at t with maturity y. In
Section 4, i equaled i(t+90) and i* equaled i*(t+90).

CIP

There is significant experimental help for CIP. See for instance Akram,
Farooq and Sarno (2008). CIP says that F(t+y)/S(t) = [1+i(t+y)]/[1+i*(t+y)],
where S(t) is the spot conversion standard and F(t+y) is the forward rate at t for
t+y as in APPP above. CIP is typically communicated in a logarithmic
guess as f(t+y) – s(t) = i(t+y) – i*(t+y).

Monetary business sectors

CIP is an illustration of the ALOP in monetary business sectors where all costs are
sell off costs. Assume f(t+y) rises to s(t), yet i*(t+y) is not exactly i(t+y).
Overlooking exchange costs, there are without risk benefits. Huge currency market
banks get 1,000,000 euro at i*(t+y), utilize that million euro to purchase 1,000,000
dollars, contribute that million bucks at the higher i(t+y) and sell those dollars
forward for euros, acquiring a practically immediate gamble free benefit of
€1,000,000.00[1+i(t+y)] short €1,000,000.00[1+i*(t+y)]. As Akram, Farooq
what’s more, Sarno (2008) bring up, in monetary sale markets open doors for
such benefits don’t endure significantly longer than a couple of moments.

In the wake of representing the different exchange costs and the way that
wares demand investment on the way, we would anticipate that exchange should be as
viable in item advertises as in monetary business sectors. How could dealers
in one market disregard sans risk benefits that merchants in another market don’t?
The typical understanding of CIP is that i(t+y) – i*(t+y) + s(t) decides
f(t+y). That translation is sensible in light of the fact that the volume of exchanges
in spot unfamiliar trade markets is more noteworthy than in any person forward
market. In any case, that translation is less persuading when we think about the joined volume of exchanges in all forward business sectors to the volume in the
spot market.
Eq. (5) is a total variant of CIP where every development is weighted
by the overall volume of exchanges in forward business sectors, wy. To the extent that we
know, nobody has at any point communicated CIP along these lines.
s(t) = wy{f(t+y) – [i(t+y) – i*(t+y)]}

APPP

The following stage to ACTFX adds the job of closeout ware markets
where, disregarding edges, f(t+y) = π(t+y) – π*(t+y). Utilizing APPP, supplant
f(t+y) in eq. (5) with π(t+y) – π*(t+y). That substitution
produces eq. (6), an ACTFX without limits.
s(t) = wy{π(t+y) – π*(t+y) – [i(t+y) – i*(t+y)]}

ACTFX portrays how the association

ACTFX portrays how the association between closeout markets for
monetary resources, i(t+y) – i*(t+y), and sell off business sectors for products,
π(t+y) ─ π*(t+y), influences spot trade rates through exchange. For APPP
alone, i.e., wy π(t+y) – π*(t+y) alone, to decide spot trade rates,
wy[i(t+y) – i*(t+y)] should be zero. For monetary business sectors alone, i.e.,
wy[i(t+y) – i*(t+y)], to decide spot trade rates, wyπ(t+y) – π*(t+y)
should be zero. That last condition can assist with making sense of why the resource approach
to trade rates comes up short.

Two benefits of eq.

Two benefits of eq. (6) are that it ought to hold for levels as well as
changes since it doesn’t utilize value records and that information ought to be
accessible consistently. There likewise is no great explanation to excuse short-run
ACTFX wild.
Eq. (6) is straightforwardly pertinent just for those nations with proper
closeout markets. That necessity limits it to created nations and
not to every single created country. Be that as it may, the financial matters behind eq. (6) applies to
all nations. At the retail level all merchandise are non-exchanged. Exchange is intriguing at
the discount level and routine just in sell off business sectors. Likewise, exchanging
wares includes time on the way.

6. Puzzles.

In Rogoff (1996), The Purchasing Power Parity Puzzle, the puzzle is the
very high short-run volatility of real exchange rates combined with the very
slow rate at which the half-lives for deviations from Purchasing Power Parity
die out. His explanation is that, in spite of progress, international commodity
markets remain highly segmented. When Rogoff refers to international
commodity markets being highly segmented, he means retail commodity
markets.

The earlier distinction between retail, wholesale and auction markets
provides a better explanation. By their very nature, international retail
markets are highly segmented and always will be because of their high transaction costs. But international auction markets are highly integrated
and have been for a long time.
In the years since 1996, the puzzles have increased and been refined.
Rogoff’s puzzle has become three related puzzles: “excessive” exchange
rate volatility, short-run versus long run and long half-lives for deviations.
Two additional puzzles are that Purchasing Power Parity appears to work
during inflation, but not in normal times, and the lack of any fundamentals
that explain the behavior of exchange rates.
The following subsections take up these puzzles in the following order:
Purchasing Power Parity works when there is inflation, but not in normal
times, It may work in the long run, but not in the short run, Long halflives for real Purchasing Power Parity differentials, (4) Exchange rate
volatility is excessive, A lack of fundamentals.

Inflation versus normal.

Frenkel (1981) is a fundamental wellspring of the possibility that PPP works during
expansion however flops in typical times. Utilizing discount and typical cost for most everyday items cost
files, he thinks about the exhibition of Buying Power Equality during the
inflationary 1920s to its exhibition during the “ordinary” 1970s. His outcomes
for discount and average cost for many everyday items files are comparative. That’s what he infers
Buying Power Equality worked during the inflationary 1920s, yet all at once fizzled
during the more ordinary 1970s.

Davutyan and Pippenger (1985)

Davutyan and Pippenger (1985) bring up that his decision is a
factual deception because of edges. A basic model comes to their meaningful conclusion.

Suppose CPPP is essentially constant and exchange rates never exceed the
thresholds. CPPP always holds, but –
2s are close to zero and regression
coefficients imprecise because within the wide thresholds there is no link
between relative prices and exchange rates.
Now consider the case where CPPP and exchange rates both rise due
to inflation and exchange rates often exceed the thresholds. CPPP often
fails, but –
2s are much larger and coefficients more precise. In the presence
of thresholds, regressions must be interpreted carefully.
This puzzle is primarily the result of mixing retail prices with auction
exchange rates in the context of thresholds. In normal times CPPP volatility
is small due to sticky retail prices and thresholds are very wide because at
retail all goods are non-traded. Wholesale prices are less sticky and
thresholds narrower, but empirically they do only slightly better in normal
times.

As expansion expands, retail and discount costs become more
adaptable. Limits are less significant. In out of control inflation those costs
turn out to be entirely adaptable and limit impacts generally vanish.
With closeout costs, the contrast among inflationary and ordinary
It would be ideal for times to a great extent vanish. Regardless of expansion, sell off costs are
entirely adaptable and limits somewhat restricted. The issue with –
to a great extent
vanishes and with it the evident qualification among inflationary and
typical times.

Long run versus short run.

The proof obviously dismisses relative CPPP for the short-run. However, there
is some help for it as a long-run hypothesis. See for instance Sarno and
Taylor (2002b) and Taylor (2006).
The answer for this puzzle is basically equivalent to for Expansion
versus Ordinary. Supplant “Expansion” with “long run” and “Ordinary” with “short
run”. Relative CPPP flops in the short run in light of the fact that tacky retail costs, time in
travel and extremely wide edges disengage spot trade rates from spot
retail costs. Discount costs improve. Over the long haul, retail and
discount costs become more adaptable and edges smaller, delivering
all the more lengthy run help for PPP.
With forward closeout costs, the contrast between short run and long
It would be ideal for run to generally vanish. In both the short run and long run, closeout
costs are adaptable and limits thin in light of the fact that data and
exchange costs per dollar are low.

Long half-lives.

Obstfeld and Rogoff (2000) list long half-lives for real CPPP differentials
as one of the six major puzzles in international macroeconomics. Wholesale
prices reduce half-lives, but they remain long.
Again, the primary sources of the problem are sticky prices and wide
thresholds combined with volatile exchange rates. Half-lives using CPPP are
very long because most tests use prices from retail markets where all goods are non-traded. It should not be a surprise that real price differentials
between non-traded goods have half-lives measured in years.
Half-lives using APPP should be much shorter. Auction prices are far
more flexible and thresholds are much narrower because information and
transaction costs per dollar are much smaller in auction markets where
commodities are traded by the shipload rather than by the pound or ounce.19
APPP indexes do not yet exist. But comparing CLOP and ALOP
provides some insight into what we can expect. As pointed out above, the
evidence rejects CLOP. But the evidence supports ALOP. As Pippenger
(2016) reports, real half-life differentials between commodity auction prices
are measured in just a few weeks despite the fact that ALOP does not hold
for spot auction markets.

Excessive volatility

As is well known, the volatility of exchange rates is much larger than
the volatility of corresponding CPPP. This difference in volatility is the
primary evidence behind the belief that exchange-rate volatility is
“excessive”. Again, a major source of the problem is mixing sticky retail
prices with volatile auction prices

Exchange rates between the U.S. and Canada

Exchange rates between the U.S. and Canada have been floating for
over 25 years. As an example of “excessive” volatility with CPPP, using
monthly data from 1975 through 2020, the variance of the change in the log
of the Canadian price of U.S. dollars is 0.000226 while the variance in the change in the log of the corresponding CPPP using consumer price indexes is
only 0.000018, a ratio of over 12 to 1.20 Exchange rate volatility is 12 times
greater than CPPP volatility.

Exchange rates are from auction market

The explanation for this puzzle is similar to the one for the three
previous puzzles. Exchange rates are from auction markets while
commodity prices are from retail markets. We are unaware of any articles
comparing the volatility of relative wholesale price indexes to the volatility of
exchange rates.
No one should be surprised to find that the volatility of the price of a
common variety of wheat on the Chicago Board of Trade, whose price
changes from minute to minute, is 12 times greater than the volatility of the
price of bread in Chicago grocery stores, whose price often does not change
for days. Why are we surprised by a ratio of 12 to 1 when we compare
auction exchange rates to relative retail price levels?

We do not yet have data for APPP, but we do have data for individual
auction commodity markets, which can give us some insight into APPP. At
least it compares auction to auction. Using weekly data from spot auction
markets, Bui and Pippenger (1990) find that the volatility of spot exchange
rates implied by spot relative prices, e.g. [($/W)0\(€/W)0], is slightly greater
than the volatility of actual spot exchange rates.21 Instead of 12 to 1, the
ratio is about 1.

Of course, their results apply to spot auction markets, not forward
auction markets. In addition, they use individual auction prices, not indexes.
But their results suggest that using APPP rather than CPPP would greatly
reduce, if not eliminate, the primary evidence for excessive volatility.

Exchange-rate disconnect.

The swapping scale separate alludes to the absence of any unmistakable connection
between trade rates and financial essentials. It is one of the six
significant riddles in Obstfeld and Rogoff (2000). ACTFX can possibly
address this riddle.
Relaxed perception recommends that overall cost levels and monetary
markets are two significant basics. CPPP fizzles for the reasons
examined previously. Why the resource way to deal with trade rates comes up short isn’t yet
self-evident, potentially in light of the fact that it overlooks relative cost levels.
Utilizing sell off business sectors for resources and wares, ACTFX consolidates
relative product cost levels and monetary business sectors. It can possibly
resolve the conversion standard disengage by connecting trade rates to monetary
furthermore, product markets. Just cautious examination can decide if or
not that potential is understood. Regardless of whether it is understood, ACTFX might be a
scaffold to a more profound comprehension of the connections among basics and
trade rates.

Collecting the data necessary to compare the CLOP and CPPP to the
ALOP and APPP will take time and be expensive. Is the game worth the
candle? The ability of APPP and/or ACTFX to explain so many puzzles
suggests that the game is worth the candle.

Summary and Conclusions.

Data and exchange costs assume significant parts in conversion scale
financial aspects. They are the wellspring of market blemishes, tacky costs and
purported “non-exchanged” products like hair styles. Yet, ordinary conversion standard
financial aspects overlooks one more impact of such expenses: the division of business sectors into
retail, discount and closeout. That division has somewhere around two significant
suggestions: (At the retail level all products, not simply hair styles, are nontraded. Subsequently, the traditional dismissal of the Law of One Cost and
Buying Power Equality, which depends essentially on retail costs, is
inappropriate, Looking at the way of behaving of tacky retail costs to the
conduct of adaptable sale trade rates makes an irrelevant comparison and
it is the wellspring of a few riddles in traditional swapping scale financial matters
examined previously.

International trade involves

Conventional exchange-rate economics also ignores the fact that, for
commodities, international trade involves time in transit. Time in transit
means that the Law of One Price and the most common version of
Purchasing Power Parity, which is based on the LOP, cannot hold for spot
commodities as is assumed in conventional tests of the LOP and PPP. As displayed above, with powerful exchange, the Law of One Cost and Buying
Power Equality hold at forward item costs and trade rates from
sell off business sectors.
Because of issues with the way regular conversion scale
financial aspects tests the Cut and PPP, this paper contends that the Hack and PPP
ought to be renamed as “not dismissed” It likewise recommends a better approach for
pondering the Hack and PPP in light of time on the way and closeout costs
that we call ALOP and APPP. ALOP and APPP tackle a few of the riddles
related with traditional swapping scale financial aspects.
Time on the way and the qualification between retail, discount and closeout
showcases likewise recommend a hypothesis of trade rates that we foster here for
whenever based successful exchange first in closeout markets for wares
what’s more, resources. We call it ACTFX. ACTFX gives a possible connection between
trade rates and basics, and a possible strong starting point for openeconomy full scale models.

Testing the general benefits of ALOP and APPP versus customary Trim
also, PPP and looking at the overall benefits of the resource way to deal with spot
trade rates versus ACTFX sets out many open doors for future
research.

APPENDIX

In Segment 4 CC$90($/W)90 is the expense of putting away W in Bay ports and
CC€90(€/W)90 is the expense of putting away W in Rotterdam, the main in future $ the
second in future €. TC$90(€/W)90($/€)90 is the expense of delivery a unit of W
from a Bay port to Rotterdam and TC€90($/W)90(€/$)90 depicts the expense of
delivering a unit of W from Rotterdam to a Bay port, the primary in future $ and
the second in future €. These capacity and transportation costs stow away the
intricacy of the edges.
In this Reference section the expense of putting away a unit of W in Bay ports is C$90 and
C€90 is the expense of putting away a unit of W in Rotterdam, the main in future $ the
second in future €. The expense of transport for a unit of W from Bay ports to
Rotterdam is T$90 and the expense from Rotterdam to Inlet ports is T€90, the first
in future $ and the second in future €. As in Segment 4, they are exogenous.
Bay to Rotterdam. THE LAW OF ONE PRICE and PPP.
Equilibria: [($/W)90-(C$90)]=($/W)0(1+ I) and (€/W)90($/€)90-(T$90)=($/W)0(1+i)
Hence [($/W)90-(C$90)]= (€/W)90($/€)90-(T$90).

Rotterdam to Bay.
Equilibria: (€/W)90-(C€90)=(€/W)0(1+ i*) and ($/W)90(€/$)90-
(T€90)=(€/W)0(1+i*).
Subsequently (€/W)90-(C€90)=($/W)90(€/$)90-(T€90).
Edges.
($/W)90/(€/W)90 = ($/€)90 – [(T$90) – (C$90)]/(€/W)90
[($/W)90/(€/W)90] = ($/€)90 + [(T€90)- (C€90)][($/€)90/(€/W)90]

As in Section 4, in the absence of carrying and transportation costs, the
exogenous exchange rate determines relative prices. As in Section 4,
carrying and transportation costs create thresholds, but here prices and
exchange rates explicitly affect thresholds.

References

Akram, Q. Farooq, D.R. and Sarno L. (2008) Arbitrage in the Foreign
Exchange Market: Turning on the Microscope. Journal of International
Economics, 76, 237-253.
Alchian, A. 1959. Costs and Outputs in M. Abramovitz et al, The
Allocation of Economic Resources, Stanford University Press, Stanford CA.
Asplund, M., Friberg, R. (2001) The Law of One Price in Scandinavian
Duty-Free Stores. American Economic Review, 91, 1072-1083.

Benninga, S., Protopapadakis, A. (1988) The Equilibrium Pricing of
Exchange Rates and Assets when Trade Takes Time. Journal of International
Money and Finance, 7,129-149.
Black, J., Hashimzade, N., Myles, G. (2012) A Dictionary of Economics.

Oxford University Press

Oxford University Press the edition, Oxford, Oxford University Press.
Bui, N., Pippenger, J. (1990) Commodity prices, exchange rates and
their relative volatility. Journal of International Money and Finance, 9(1), 3-
20. A Model of Spatial Arbitrage with Transport
Capacity Constraints and Endogenous Transport Prices. American Journal of
Agricultural Economics, 91(1), 42-56.
Coleman, A. (2009b) Storage, Slow Transport, and the Law of One
Price: Theory with Evidence from Nineteenth-Century U.S. Corn Markets. The
Review of Economics and Statistics, 91(2), 332-350.  THE LAW OF ONE PRICE and PPP.

Davutyan, N., Pippenger, J. (1985) for THE LAW OF ONE PRICE and PPP

Davutyan, N., Pippenger, J. (1985) Purchasing Power Parity Did Not
Collapse During the 1970s. The American Economic Review. 75(5), 1151-
1158.
Engel, C., Rogers, J.H. (1996) How Wide Is the Border? American
Economic Review, 86, 1112-1125.
Frenkel, J.A. (1981) The Collapse of Purchasing Power Parities during
the 1970s. European Economic Review, 16, 145-65.

Goodwin, B.K., (1992) Cointegration Tests of the Law of One Price in
International Wheat Markets. Review of Agricultural Economics, 14, 117-
124.
Hempel, C.G. (1966) Philosophy of Natural Science, Prentice-Hall, Inc.,
Englewood Cliffs, N.J.
Isard, P. (1995) Exchange Rate Economics, Cambridge University
Press, Cambridge, England.
Kargbo, J.M. (2009) Financial globalization and purchasing power parity
in the G7 countries. Applied Economics Letters, 16(1), 69-74. Kim, Y. (1990) Purchasing Power Parity: Another Look at the Long-run
Data. Economic Letters, 32(4), 339-344.

Kouretas, G.P. (1997) for THE LAW OF ONE PRICE and PPP

Kouretas, G.P. (1997) The Canadian Dollar and Buying Power Equality
during the New Float. Survey of Worldwide Financial matters, 5(4), 467-477.
Michael, P., Nobay R., Strip, D. (1994) Buying power equality yet
once more: proof from spatially isolated ware markets. Diary of
Worldwide Cash and Money, 13(6), 637-657. THE LAW OF ONE PRICE and PPP.

Obstfield, M., Rogoff, K. (2000) The Six Significant Riddles in Worldwide
Macroeconomics: Is There a Typical Reason? NBER Macroeconomics Yearly,
15, 339-390.

Official, L. (1976) The Buying – Power-Equality Hypothesis of Trade
Rates: A Survey Article. IMF Staff Papers, 23, 1-60.

Parsley, D.C., Wei, SJ. (2001) Making sense of the boundary impact: the job of
conversion scale changeability, transporting expenses and geology. Diary of
Worldwide Financial aspects, 55, 87-105.
Pippenger, J. (2016) Product Exchange and the Law of One Cost:
Clearing up everything. Hypothetical Financial Letters, 6, 1017-1033.

Rogoff, K. (1996) The Buying Power Equality Puzzle. Diary of
Financial Writing, 34(2), 647-668.
Sarno, L. (2005) Perspective: Towards an answer for the riddles in
conversion scale financial aspects: what’s the situation? Canadian Diary of
Financial matters, 38(3), 673-708.
Sarno, L., Taylor, M.P. (2002a) The Financial matters of Trade Rates,
Cambridge College Press, Cambridge, Britain.
Sarno, L., Taylor, M.P. (2002b) Buying Power Equality and the Genuine
Conversion scale. IMF Staff Pappers, 49(1), 65-105.
Taylor, M.P. (2006) Genuine trade rates and Buying Power Equality:
mean-inversion in financial idea. Applied Monetary Financial matters, 16(1-2),
1-17.

Winther, Rasmus Grøfeldt. 2016 Winter version. The Design of
Logical Speculations, E.N. Zalta Ed., The Stanford Reference book of Reasoning,
Stanford College, Stanford, CA.